Effects of Intraspecific Competition Delay on Vegetation Periodic Oscillation Patterns
-
摘要:
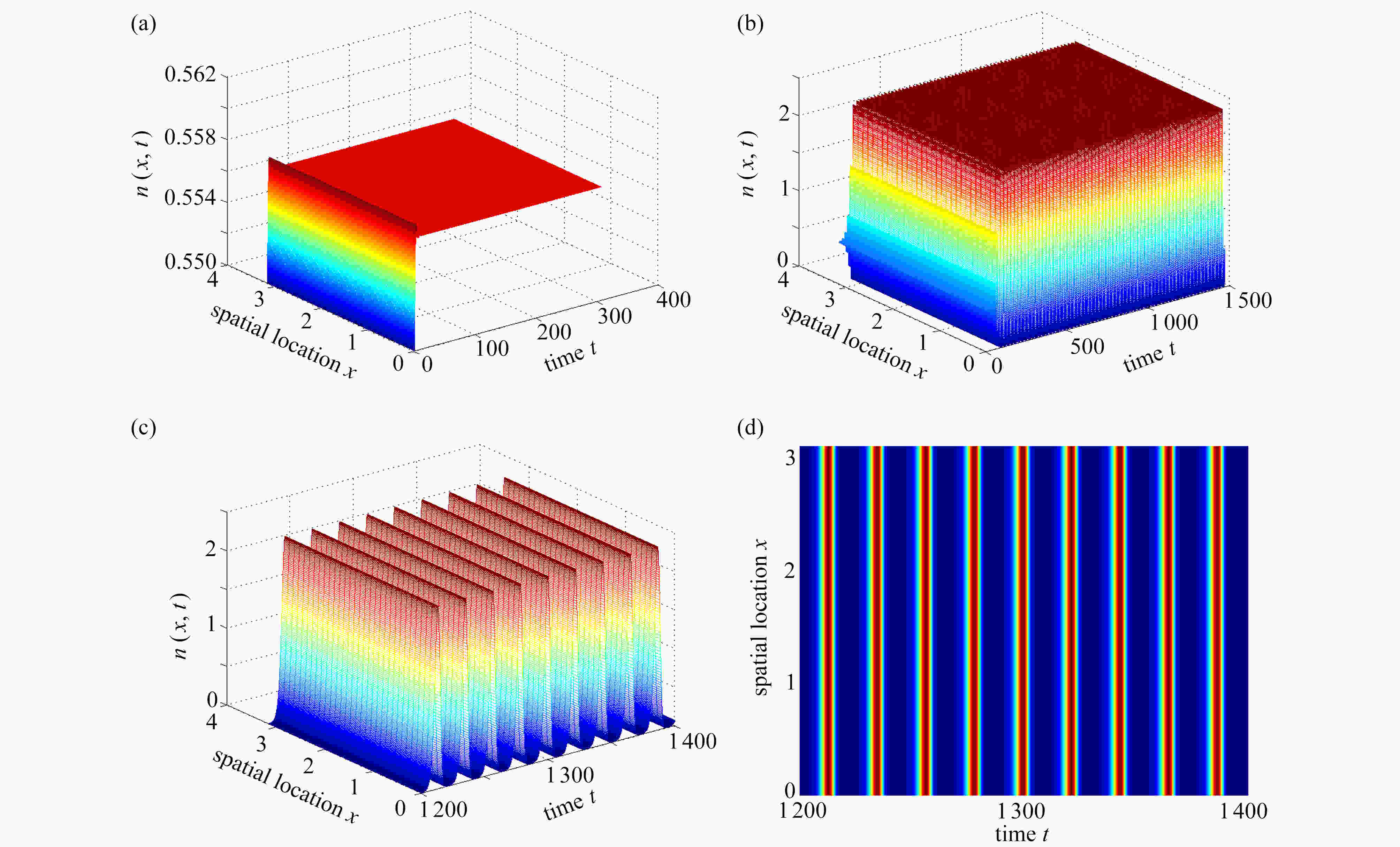
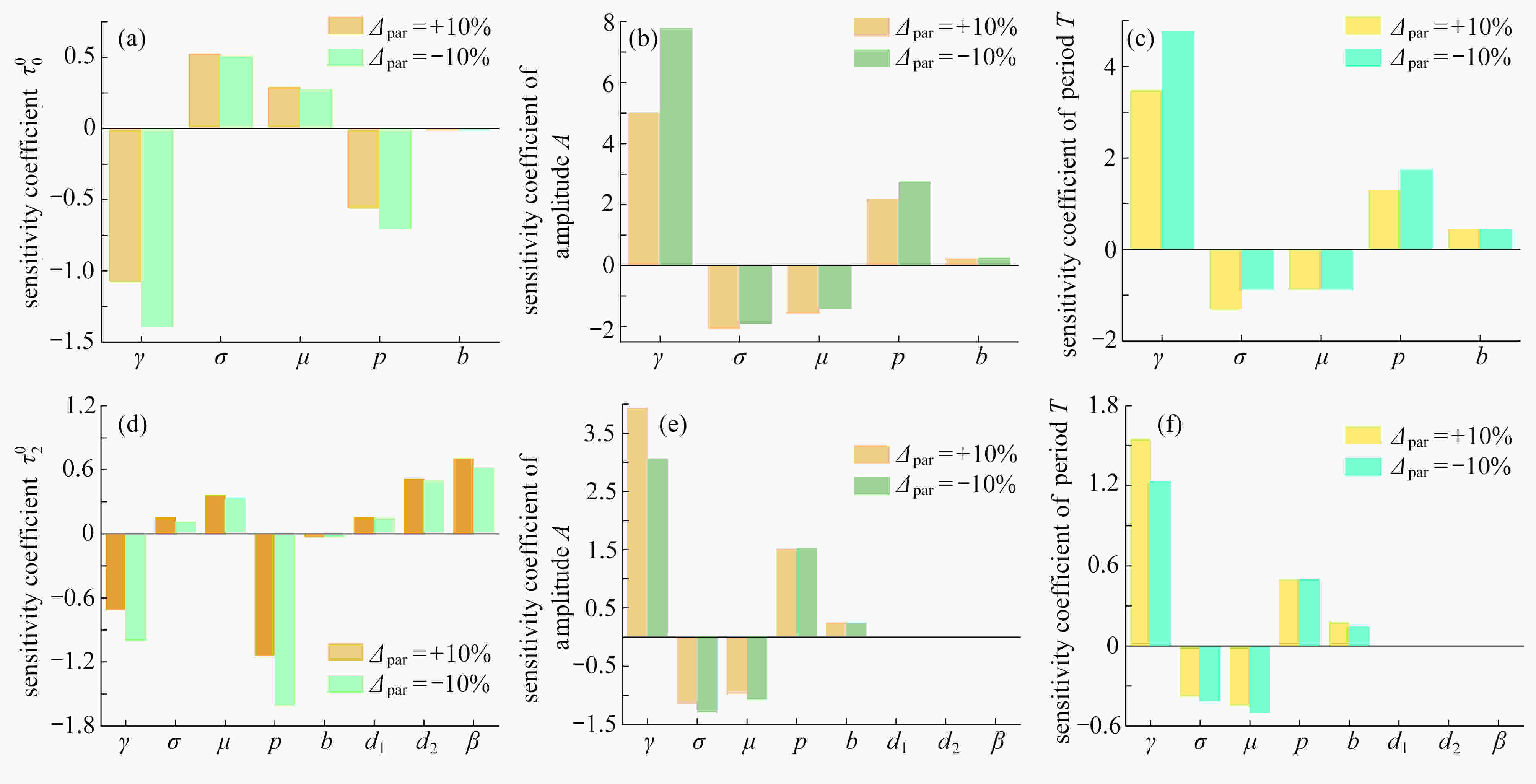
考虑到干旱、半干旱地区的幼年植被与成年植被之间存在竞争水资源的现象,该文构建一个具有种内竞争时滞的植被-土壤水动力学模型。分析出系统存在植被灭绝平衡点和植被生存平衡点,并给出了平衡点局部稳定的条件,分别给出非空间系统和空间系统产生Hopf分支周期解的条件。通过数值模拟展示出两种系统对应的植被随时间演化做周期振荡模式,并通过参数敏感性分析发现降雨量和植被的增长率对这种模式的产生和模式的振幅、周期有显著影响,但蒸发量的影响效果最不显著,表明降雨量和植被本身的特征对干旱、半干旱地区植被的演化发展产生了深刻的影响。同时发现空间扩散的引入会抑制这种模式的发生,但对振幅和周期没有任何影响。所获得的结果解释了自然界中广泛观察到的植被周期振荡现象,为植被系统的可持续发展提供了理论支撑。
Abstract:Aimed at the phenomenon of competing for water resources between young vegetation and adult vegetation in arid and semi-arid areas, a vegetation-soil water dynamic model with intraspecfic competition delay was established. The conditions for the existence of an unique vegetation survival equilibrium and the local stability of the vegetation extinction equilibrium were analyzed. The generating conditions for Hopf bifurcating periodic solutions of non-spatial and spatial systems were given, respectively. The periodic oscillation pattern appearing in the vegetation evolution with time was numerically simulated. Through the parameter sensitivity analysis, the rainfall and the vegetation growth rate were found to have significant influences on the generation, the amplitude and the period of this pattern, while the effects of evaporation was found to be the least significant. The results indicate that, the rainfall and the vegetation properties have profound impacts on the evolution and development of vegetation in arid and semi-arid areas. The introduction of spatial diffusion inhibits the occurrence of this pattern, but doesn’t affect the amplitude and the period. The work explains the phenomenon of vegetation periodic oscillation widely observed in nature, and provides theoretical supports for the sustainable development of the vegetation system.
-
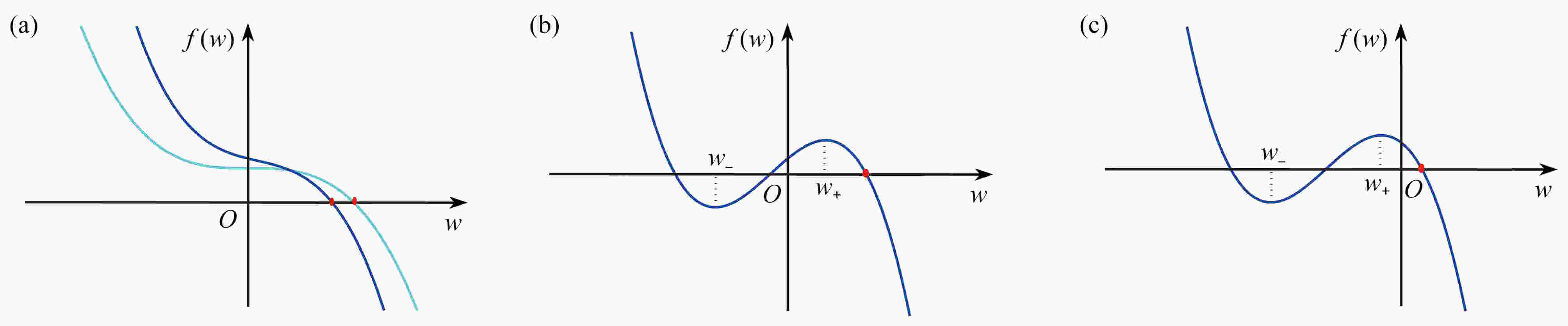
图 2 方程(4)存在唯一正实数根的示意图:(a) 情形(Ⅰ);(b) 情形(Ⅱ)(i);(c) 情形(Ⅱ)(ii)(其中红色实心点代表正实数根,图(a)中亮蓝色和蓝色曲线分别表示
$\varDelta=0$ 和$\varDelta < 0$ 的情形)注 为了解释图中的颜色,读者可以参考本文的电子网页版本,后同。
Figure 2. Schematic diagrams of the existence of the unique positive real root for eq. (4): (a) case (Ⅰ); (b) case (Ⅱ)(i); (c) case (Ⅱ)(ii), where the red dot represents the positive real root, the light blue and the blue curves of fig. (a) represent the cases of
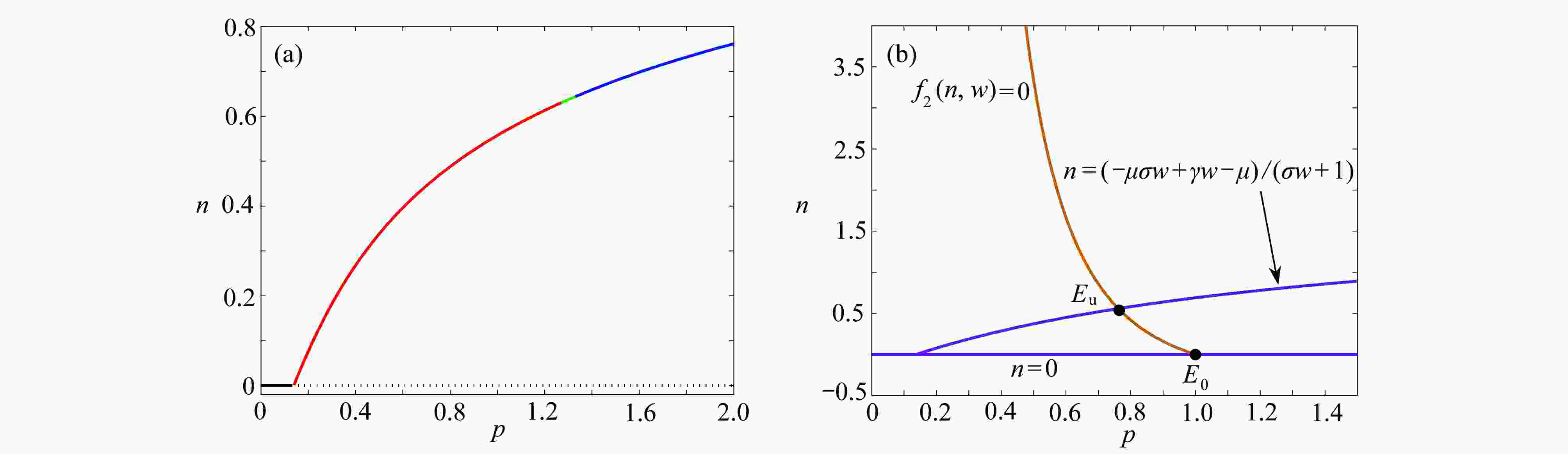
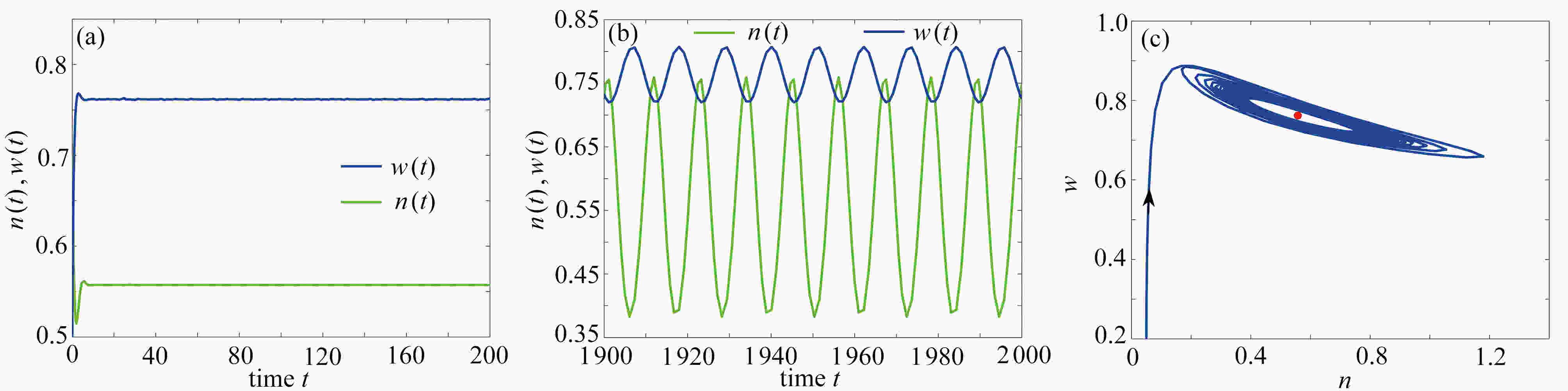
$\varDelta=0$ and$\varDelta < 0$ , respectively图 3 平衡点的存在情况和零斜率线图: (a) 植被生物量
$n$ 随着参数$p$ 的变化;(b) 在w-n平面上零斜率线$f_{1}(n,w)=0$ (紫色/洋红色)和$f_{2}(n,w)=0$ (橘色)形成的交点(黑色实心点),其中$E_{0}$ 和$E_{{\rm{u}}}$ 表示植被灭绝平衡点和植被生存平衡点,参数$\gamma=1.6$ ,$\sigma=0.8$ ,$\mu=0.2$ ,$b=0.2$ Figure 3. The existence of equilibria and zero-slope diagrams: (a) vegetation biomass
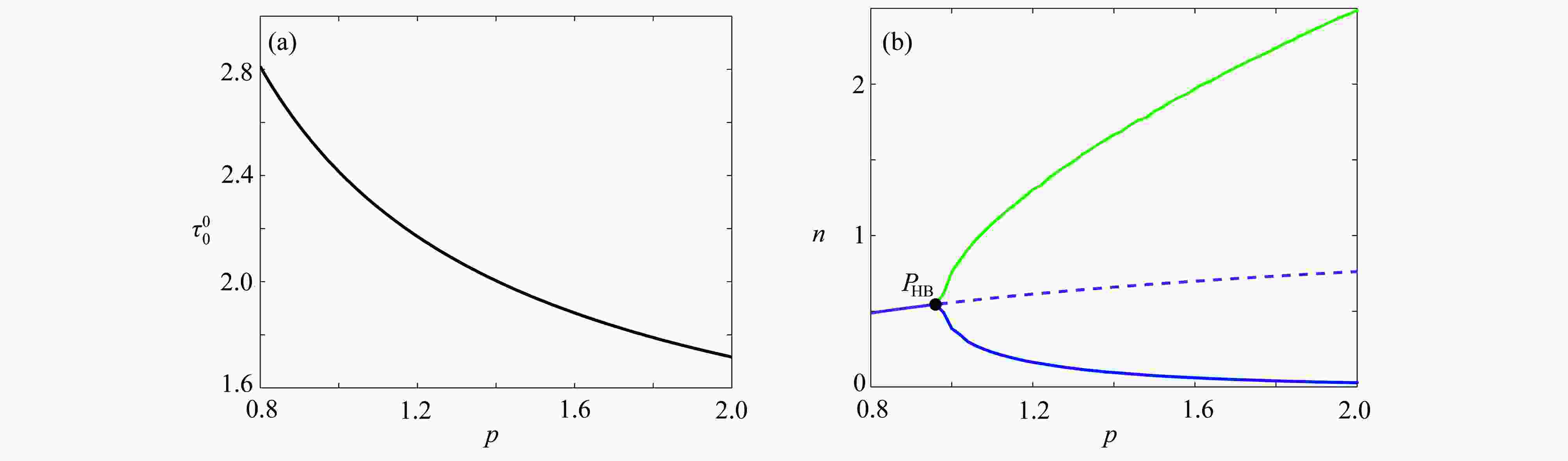
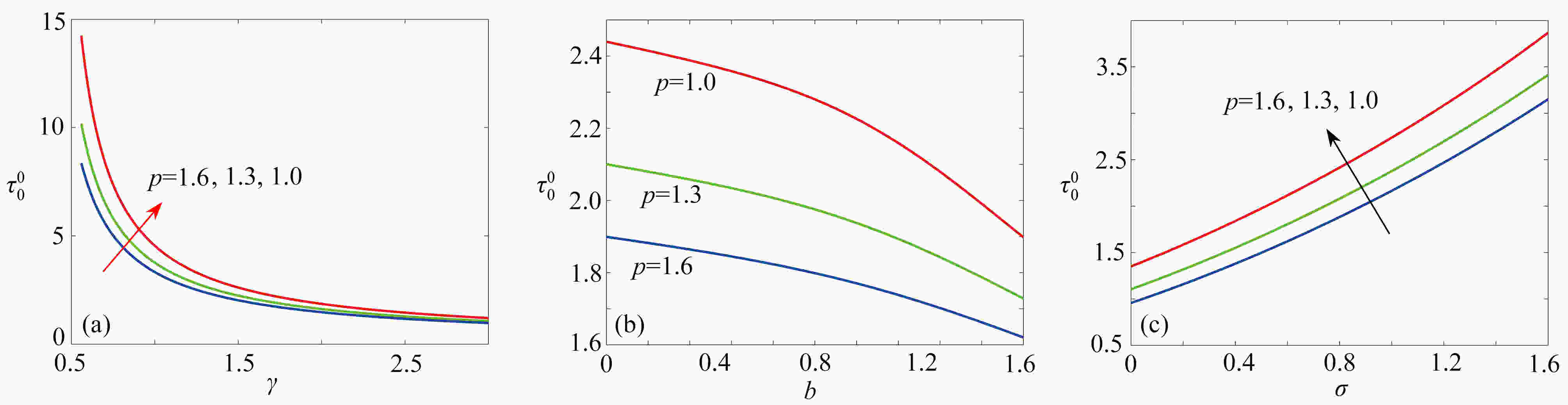
$n$ vs.$p$ ; (b) the intersection (black solid points) formed by zero-slope line$f_{1}(n,w)=0$ (purple/magenta) and$f_{2}(n,w)=0$ (orange) on$w{\text{-}}n$ plane, where$E_{0}$ and$E_{{\rm{u}}}$ are the vegetation extinction equilibrium points and the vegetation existence equilibrium points,$\gamma=1.6$ ,$\sigma=0.8$ ,$\mu=0.2$ ,$b=0.2$ 图 4 临界时滞
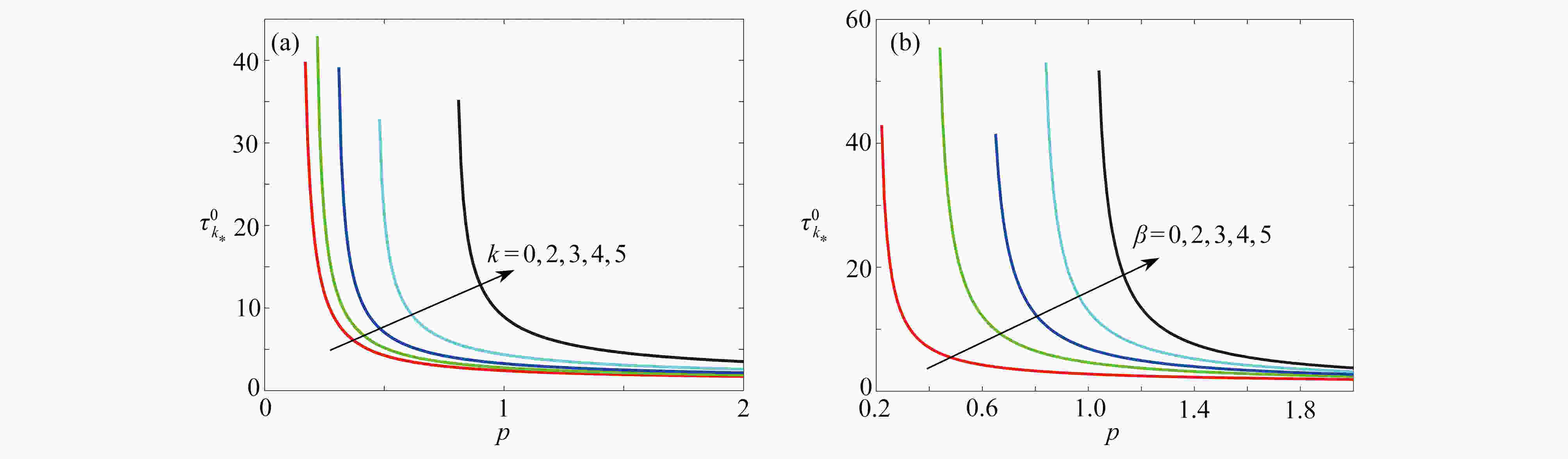
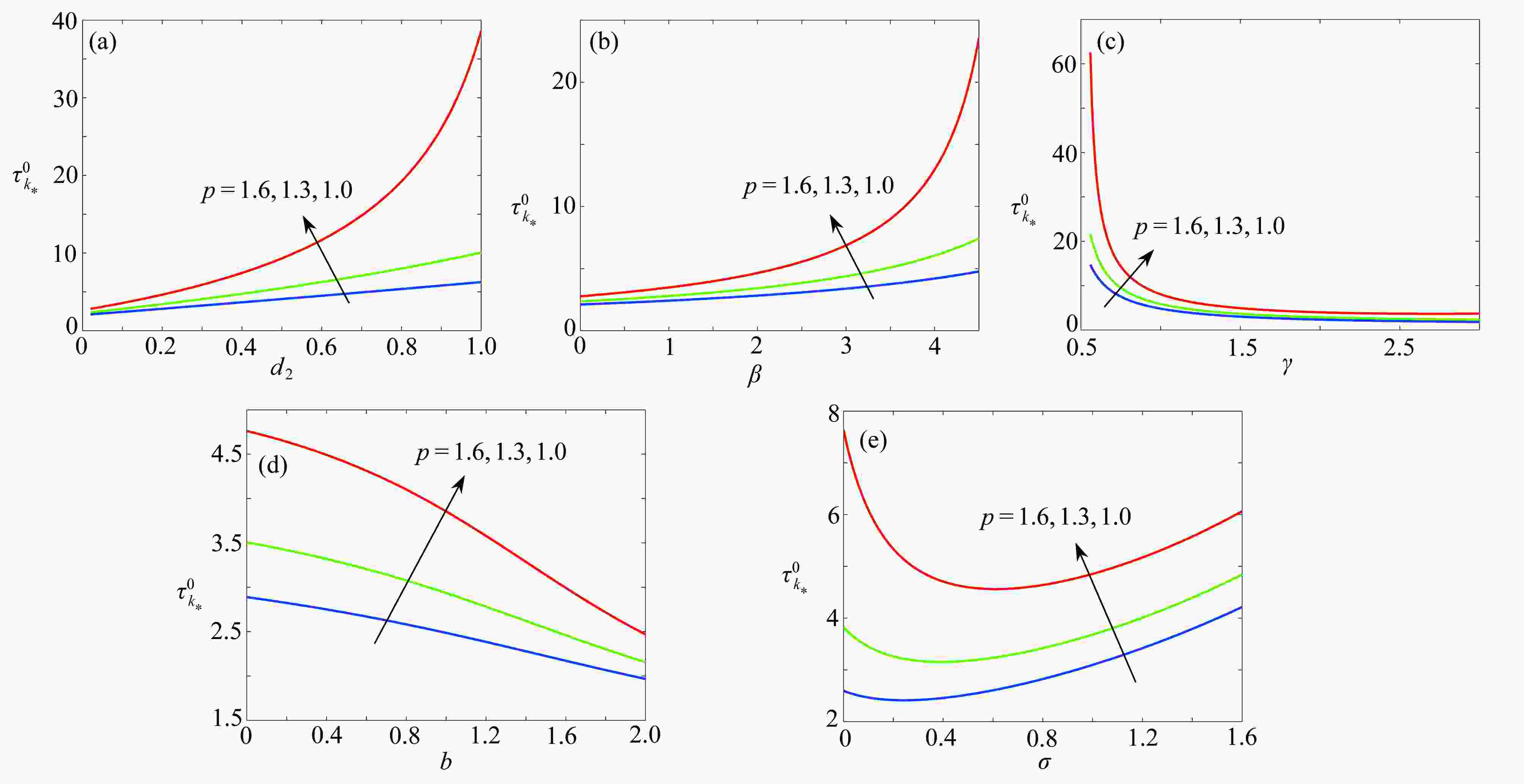
$\tau_{0}^{0} $ 和植被生物量随参数p的变化情况: (a)$\tau_{0}^{0}$ 随参数$p$ 的变化情况;(b)$(p,n)$ 平面上的单参数分支图,参数$\sigma=0.8$ ,$\mu=0.2$ ,$b=0.2$ ,$\gamma=1.6$ ,$\tau=3$ Figure 4. The changes of the critical delay
$\tau_{0}^{0} $ and the vegetation biomass with parameter p: (a) the variation of$\tau_{0}^{0}$ with$p$ ; (b) bifurcation diagram in$(p,n)$ plane for$\sigma=0.8$ ,$\mu=0.2$ ,$b=0.2$ ,$\gamma=1.6$ and$\tau=3$ 表 1 系统(3)正平衡点的存在情况
Table 1. Existence properties of positive equilibrium points for system (3)
existence condition equilibrium point $\gamma-\mu\sigma \leqslant 0$ $E_{0}$ $\gamma-\mu\sigma > 0 :\;\varDelta \leqslant 0$ $E_{0}$,$E_{*}$ $\gamma-\mu\sigma > 0 :\; \varDelta \gt 0$,$\alpha_{1}>0$ $E_{0}$,$E^{*}$ $\gamma-\mu\sigma > 0 :\; \varDelta \gt 0$,$\alpha_{1}<0$,$\alpha_{2}<0$ $E_{0}$,$E_{1}$ -
[1] EIGENTLER L, SHERRATT J A. Metastability as a coexistence mechanism in a model for dryland vegetation patterns[J]. Bulletin of Mathematical Biology, 2019, 81(7): 2290-2322. doi: 10.1007/s11538-019-00606-z [2] PRINGLE R M, DOAK D F, BRODY A K, et al. Spatial pattern enhances ecosystem functioning in an African savanna[J]. PLoS Biology, 2010, 8(5): e1000377. doi: 10.1371/journal.pbio.1000377 [3] GOWDA K, CHEN Y, IAMS S, et al. Assessing the robustness of spatial pattern sequences in a dryland vegetation model[J]. Proceedings of the Royal Society A, 2016, 472: 20150893. doi: 10.1098/rspa.2015.0893 [4] GETZIN S, YIZHAQ H, BELL B, et al. Discovery of fairy circles in Australia supports self-organization theory[J]. Proceedings of the National Academy of Sciences of the United States of America, 2016, 113(13): 3551-3556. doi: 10.1073/pnas.1522130113 [5] GILAD E, VON HARDENBERG J, PROVEBZALE A, et al. Ecosystem engineers: from pattern formation to habit creation[J]. Physical Review Letters, 2004, 93(9): 098105. doi: 10.1103/PhysRevLett.93.098105 [6] KLAUSMEIER C A. Regular and irregular patterns in semiarid vegetation[J]. Science, 1999, 284(5421): 1826-1828. doi: 10.1126/science.284.5421.1826 [7] VON HARDENBERG J, MERON E, SHACHAK M, et al. Diversity of vegetation patterns and desertification[J]. Physical Review Letters, 2001, 87(19): 198101. doi: 10.1103/PhysRevLett.87.198101 [8] LIU Q X, JIN Z, LI B L. Numerical investigation of spatial pattern in a vegetation model with feedback function[J]. Journal of Theoretical Biology, 2008, 254(2): 350-360. doi: 10.1016/j.jtbi.2008.05.017 [9] BONACHELA J A, PRINGLE R M, SHEFFER E, et al. Termite mounds can increase the robustness of dryland ecosystems to climatic change[J]. Science, 2015, 347(6222): 651-655. doi: 10.1126/science.1261487 [10] TARNITA C E, BONACHEL J A, SHEFFER E, et al. A theoretical foundation for multi-scale regular vegetation patterns[J]. Nature, 2017, 541(7637): 398-401. doi: 10.1038/nature20801 [11] SUN G Q, WANG C H, CHANG L L, et al. Effects of feedback regulation on vegetation patterns in semi-arid environments[J]. Applied Mathematical Modelling, 2018, 61: 200-215. doi: 10.1016/j.apm.2018.04.010 [12] LI J, SUN G Q, JIN Z. Interaction of time delay and spatial diffusion induce the periodic oscillation of the vegetation system[J]. Discrete and Continuous Dynamical Systems (Series B) , 2022, 27(4): 2147-2172. doi: 10.3934/dcdsb.2021127 [13] 曹建智, 谭军, 王培光. 一类具有时滞的云杉蚜虫种群模型的Hopf分岔分析[J]. 应用数学和力学, 2019, 40(3): 332-342. (CAO Jianzhi, TAN Jun, WANG Peiguang. Hopf bifurcation analysis of a model for spruce budworm populations with delays[J]. Applied Mathematics and Mechanics, 2019, 40(3): 332-342.(in Chinese)CAO Jianzhi, TAN Jun, WANG Peiguang. Hopf bifurcation analysis of a model for spruce budworm populations with delays[J]. Applied Mathematics and Mechanics, 2019, 40(3): 332-342. (in Chinese) [14] 谷雨萌, 黄明迪. 一类时间周期的时滞竞争系统行波解的存在性[J]. 应用数学和力学, 2020, 41(6): 658-668. (GU Yumeng, HUANG Mingdi. Existence of periodic traveling waves for time-periodic Lotka-Volterra competition systems with delay[J]. Applied Mathematics and Mechanics, 2020, 41(6): 658-668.(in Chinese)GU Yumeng, HUANG Mingdi. Existence of periodic traveling waves for time-periodic lotka-volterra competition systems with delay[J]. Applied Mathematics and Mechanics, 2020, 41(6): 658-668. (in Chinese) [15] 欧阳颀. 非线性科学与斑图动力学导论[M]. 北京: 北京大学出版社, 2010.OUYANG Qi. Introduction to Nonlinear Science and Pattern Dynamics[M]. Beijing: Peking University Press, 2010. (in Chinese) [16] INGALLS B. Sensitivity analysis: from model parameters to system behaviour[J]. Essays in Biochemistry, 2008, 45: 177-194. doi: 10.1042/bse0450177 [17] KÉFI S, RIETKERK M, ALADOS C L, et al. Spatial vegetation patterns and imminent desertification in Mediterranean arid ecosystems[J]. Nature, 2007, 449(7159): 213-217. doi: 10.1038/nature06111 [18] BASTIAANSEN R, JAÏBI O, DEBLAUWE V, et al. Multistability of model and real dryland ecosystems through spatial self-organization[J]. Proceedings of the National Academy of Sciences, 2018, 115(44): 11256-11261. doi: 10.1073/pnas.1804771115 -




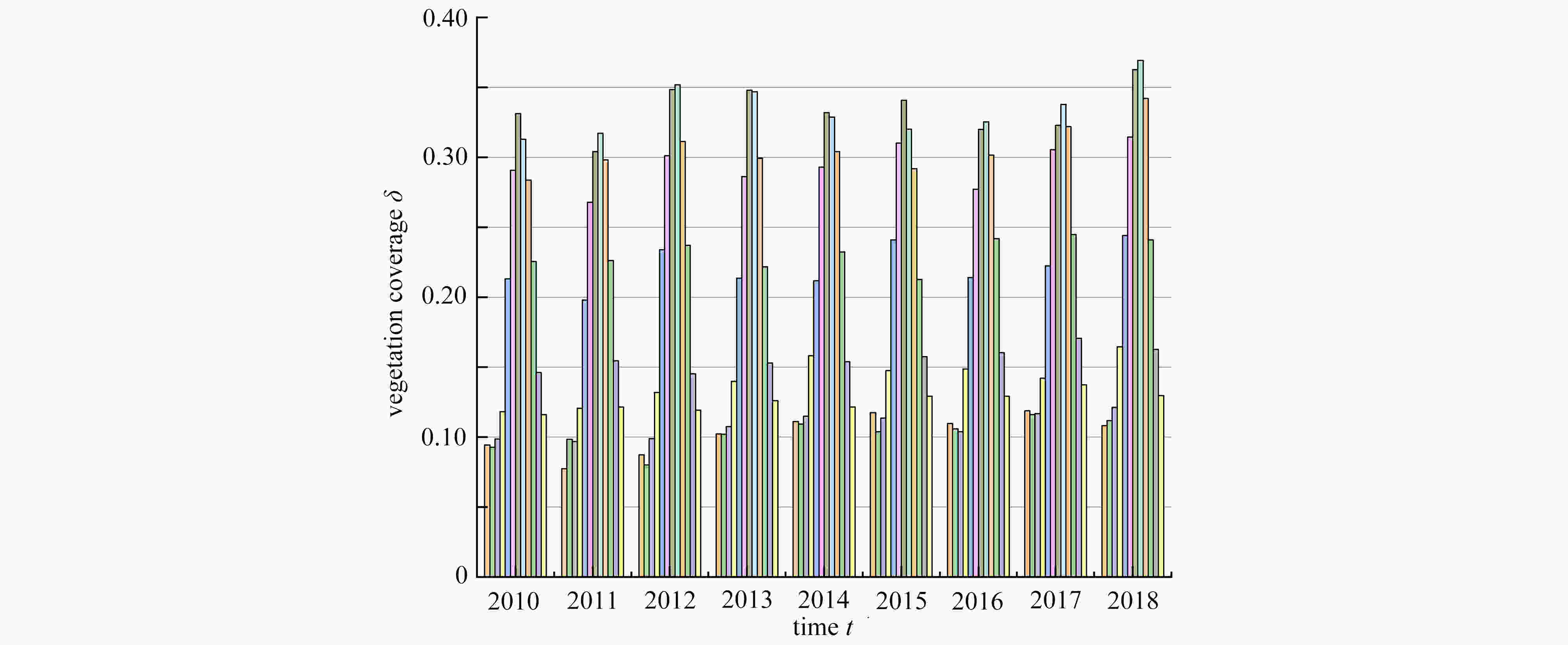
 下载:
下载:















































































































 渝公网安备50010802005915号
渝公网安备50010802005915号