Research on Anti-Seismic Performances of Interlayer Isolation Structures With Lateral Stopping Viscoelastic Dampers
-
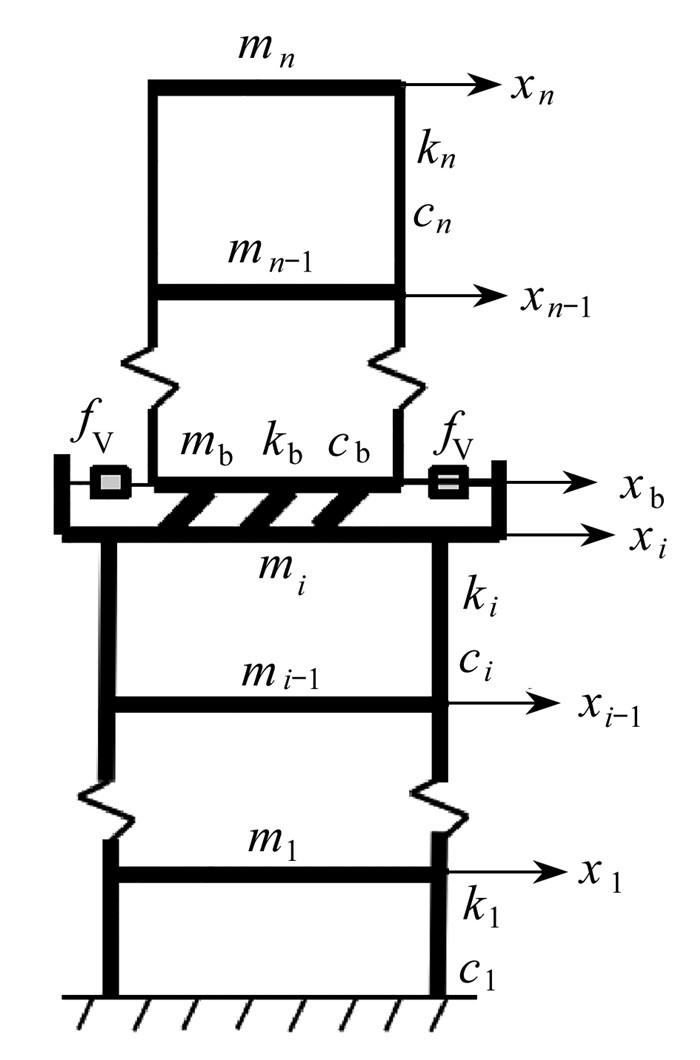
摘要: 对在隔震层设置侧向限位黏弹性阻尼器组成混合耗能结构随机激励下的减震性能进行了系统研究. 首先, 建立混合耗能结构的地震动方程,利用双过滤白噪声激励地震动的滤波方程,将该类激励下混合耗能结构的动力学问题精确转化为基于易于获得简明封闭解的白噪声激励问题. 其次,基于复模态法和白噪声激励的Dirac函数性质推导了混合耗能结构系列响应(结构位移、层间位移和阻尼器阻尼力)的方差和0~2阶谱矩的简明封闭解. 最后,通过算例在验证所提封闭解正确的基础上研究了混合耗能结构减震性能的影响因素. 研究表明:层间隔震层以上楼层的结构响应量随着隔震层刚度的增大而增大;黏弹性阻尼器阻尼参数对隔震层以上楼层的影响特征为,结构位移在一定阻尼参数下可达到最小,而层间位移则随着阻尼参数的增大而增大. 该研究可为层间隔震混合耗能结构的设计提供参考.Abstract: Anti-seismic performances of the hybrid energy dissipation structure (HEDS) composed of an isolation interlayer with lateral stopping viscoelastic dampers (VEDs) under random excitation were systematically investigated. Firstly, the seismic motion equations for the HEDS subjected to the double-filtered white noise excitation (DFWNE) were established, and the filtering equation of the DFWNE was used to accurately transform the dynamic calculation of the HEDS based on the DFWNE into white noise excitation problems to be easily tackled with concise closed-form solutions. Secondly, based on the complex mode method and the properties of the Dirac function for white noise excitation, a concise closed-form solution of the variance and 0th-to 2nd-order spectral moments of the series of responses (structural displacements, interlayer displacements, and damper damping forces) of the HEDS, was derived. Finally, based on the verification of the correctness of the proposed closed solution through numerical examples, the influencing factors on the seismic performances of the HEDS were studied. The results show that, the structural responses of floors above the isolation layer increase with the stiffness of the isolation layer; while the damping parameter of the VED have different effects on the interlayer displacement and structural displacements of floors above the isolation layer, i.e., the structural displacement can reach the minimum under certain damping parameter of the VED, but the interlayer displacement increases with the damping parameter of the VED. This study can provide reference for the design of HEDSs.
-
表 1 阻尼器出力0阶、1阶谱矩计算
Table 1. The 0th-and 1st-order spectral moments of damper's force
method Δω/(rad/s) 0th-order/N2 1st-order/(N2·s-1) the pseudo excitation method (PEM) 1 32.856 6 87.709 0.1 98.929 3 186.916 0.01 99.906 5 188.356 the proposed method 99.903 7 188.356 -
[1] 吴应雄, 黄净, 林树枝, 等. 建筑隔震构造设计与应用现状[J]. 土木工程学报, 2018, 51(2): 62-73. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC201802008.htmWU Yingxiong, HUANG Jing, LIN Shuzhi, et al. Design and application status of seismic isolation constitution of building[J]. China Civil Engineering Journal, 2018, 51(2): 62-73. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC201802008.htm [2] ZHANG R H, SOONG T T, MAHMOODI P. Seismic response of steel frame structures with added viscoelastic dampers[J]. Earthquake Engineering & Structural Dynamics, 1989, 18(3): 389-396. [3] 欧进萍, 王永富. 设置TMD、TLD控制系统的高层建筑风振分析与设计方法[J]. 地震工程与工程振动, 1994, 14(2): 61-75. https://www.cnki.com.cn/Article/CJFDTOTAL-DGGC199402005.htmOU Jinping, WANG Yongfu. Wind-induced vibration analysis and design method of tall buildings with tuned mass dampers or tuned liquid dampers[J]. Earthquake Engineering and Engineering Vibration, 1994, 14(2): 61-75. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-DGGC199402005.htm [4] 李创第, 江丽富, 王瑞勃, 等. 单自由度混联Ⅱ型惯容系统随机地震动响应分析[J]. 应用数学和力学, 2023, 44(3): 260-271. doi: 10.21656/1000-0887.430166LI Chuangdi, JIANG Lifu, WANG Ruibo, et al. Responses of SDOF structures with SPIS-Ⅱ dampers under random seismic excitation[J]. Applied Mathematics and Mechanics, 2023, 44(3): 260-271. (in Chinese) doi: 10.21656/1000-0887.430166 [5] 武田寿一. 建筑物隔震防振与控振[M]. 纪晓惠, 译. 北京: 中国建筑工业出版社, 1997.TAKEDA Shouyi. Building Isolation, Vibration Prevention, and Control [M]. JI Xiaohui, transl. Beijing: China Construction Industry Press, 1997. (in Chinese) [6] 周福霖, 张颖, 谭平. 层间隔震体系的理论研究[J]. 土木工程学报, 2009, 42(8): 1-8. https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC200908000.htmZHOU Fulin, ZHANG Ying, TAN Ping. Theoretical study on interlayer isolation system[J]. China Civil Engineering Journal, 2009, 42(8): 1-8. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TMGC200908000.htm [7] 张尚荣, 谭平, 杜永峰, 等. 层间隔震体系可靠度的灵敏度分析[J]. 振动、测试与诊断, 2016, 36(1): 102-107. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCS201601020.htmZHANG Shangrong, TAN Ping, DU Yongfeng, et al. Reliability sensitivity analysis of inter-story isolation system[J]. Journal of Vibration, Measurement & Diagnosis, 2016, 36(1): 102-107. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCS201601020.htm [8] 祁皑, 郑国琛, 阎维明. 考虑参数优化的层间隔震结构振动台试验研究[J]. 建筑结构学报, 2009, 30(2): 8-16. doi: 10.3321/j.issn:1000-6869.2009.02.002QI Ai, ZHENG Guochen, YAN Weiming. Shaking table test of interlayer isolation structure considering parameter optimization [J]. Journal of Building Structures, 2009, 30(2): 8-16. (in Chinese) doi: 10.3321/j.issn:1000-6869.2009.02.002 [9] 孙臻, 刘伟庆, 王曙光, 等. 苏豪银座层间隔震结构设计与地震响应分析[J]. 建筑结构, 2013, 43(18): 58-63. https://www.cnki.com.cn/Article/CJFDTOTAL-JCJG201318012.htmSUN Zhen, LIU Weiqing, WANG Shuguang, et al. Design and seismic response analysis of interlayer isolation structure of Suhao Ginza[J]. Architectural Structure, 2013, 43(18): 58-63. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JCJG201318012.htm [10] 刘军生, 王社良, 石韵, 等. 带限位装置的新型摩擦滑移隔震结构振动台试验研究[J]. 西安建筑科技大学学报(自然科学版), 2015, 47(4): 498-502. https://www.cnki.com.cn/Article/CJFDTOTAL-XAJZ201504007.htmLIU Junsheng, WANG Sheliang, SHI Yun, et al. New friction sliding isolation structures with limit devices [J]. Journal of Xi'an University of Architecture and Technology (Natural Science Edition), 2015, 47(4): 498-502. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XAJZ201504007.htm [11] 翁锦华. 惯容装置限位的层间隔震结构减震性能研究[J]. 应用基础与工程科学学报, 2022, 30(5): 1188-1200. https://www.cnki.com.cn/Article/CJFDTOTAL-YJGX202205011.htmWENG Jinhua. Study on seismic performance of story isolation structure with inerter device[J]. Journal of Basic Science and Engineering, 2022, 30(5): 1188-1200. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YJGX202205011.htm [12] 赵立菊, 葛新广, 王善库. 基于双过滤白噪声激励的层间隔震结构随机响应的解析解法[J]. 结构工程师, 2020, 36(4): 81-89. doi: 10.3969/j.issn.1005-0159.2020.04.012ZHAO Liju, GE Xinguang, WANG Shanku. Analytical method for random response of story isolated structures excited by double filtered white noise [J]. Structural Engineer, 2020, 36(4): 81-89. (in Chinese) doi: 10.3969/j.issn.1005-0159.2020.04.012 [13] GE X G, AZIM I, LI C, et al. Seismic responses of structure with six-element generalized model viscoelastic dampers[J]. Soil Dynamics and Earthquake Engineering, 2023, 164: 107610. doi: 10.1016/j.soildyn.2022.107610 [14] XIE LY, LI Z, CHAO P, et al. Uniform damping ratio-based design method for seismic retrofitting elastoplastic RC structures using viscoelastic dampers[J]. Soil Dynamics and Earthquake Engineering, 2020, 128: 105866. doi: 10.1016/j.soildyn.2019.105866 [15] 周继磊, 杨迪雄, 陈国海. 近断层脉冲型地震动功率谱特性分析[J]. 世界地震工程, 2017, 33(1): 18-26. https://www.cnki.com.cn/Article/CJFDTOTAL-SJDC201701003.htmZHOU Jilei, YANG Dixiong, CHEN Guohai. Characteristic analysis of power spectrum for near-fault impulse type ground motion[J]. World Earthquake Engineering, 2017, 33(1): 18-26. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SJDC201701003.htm [16] 钟庭, 陈辉国, 刘国粹, 等. 地震波双模态时变修正Kanai-Tajimi非平稳随机模型的改进及参数识别[J]. 地震工程学报, 2017, 39(1): 72-79. https://www.cnki.com.cn/Article/CJFDTOTAL-ZBDZ201701011.htmZHONG Ting, CHEN Huiguo, LIU Guocui, et al. Improvement and parameter identification of bimodal time variables modified by the Kanai-Tajimi nonstationary stochastic model using strong ground motion records[J]. China Earthquake Engineering Journal, 2017, 39(1): 72-79. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZBDZ201701011.htm [17] 李创第, 陈明杰, 葛新广. 基于Clough-Penzien谱激励的指数型非黏滞阻尼结构随机地震动响应简明封闭解[J]. 应用数学和力学, 2021, 42(3): 282-291. doi: 10.21656/1000-0887.410151LI Chuangdi, CHEN Mingjie, GE Xinguang. A simple closed response solution to random ground motion for exponential non-viscous-damping structures based on the Clough-Penzien spectrum excitation[J]. Applied Mathematics and Mechanics, 2021, 42(3): 282-291. (in Chinese) doi: 10.21656/1000-0887.410151 [18] LI J, YAN Q, CHEN J B. Stochastic modeling of engineering dynamic excitations for stochastic dynamics of structures[J]. Probabilistic Engineering Mechanics, 2012, 27(1): 19-28. doi: 10.1016/j.probengmech.2011.05.004 [19] 李创第, 丁晓华, 陈俊忠, 等. 基础隔震结构基于Clough-Penzien谱随机地震响应分析的复模态法[J]. 振动与冲击, 2006, 25(5): 162-165. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200605038.htmLI Chuangdi, DING Xiaohua, CHEN Junzhong, et al. Complex modal method for analysis of random earthquake response of structures with base isolation on the basis of Clough-Penzien spectrum[J]. Journal of Vibration and Shock, 2006, 25(5): 162-165. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200605038.htm [20] 李春祥, 熊学玉. 基于Kanai-Tajimi/Clough-Penzien模型时MTMD的动力特性[J]. 振动与冲击, 2002, 21(4): 39-43. https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200204008.htmLI Chunxiang, XIONG Xueyu. Dynamic characteristics of MTMD based on Kanai-Tajimi/Clough-Penzien models[J]. Journal of Vibration and Shock, 2002, 21(4): 39-43. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-ZDCJ200204008.htm [21] 李创第, 张翊, 葛新广. 单自由度Maxwell阻尼器耗能结构基于频响函数谱矩的等效阻尼[J]. 广西大学学报(自然科学版), 2019, 44(1): 41-51. https://www.cnki.com.cn/Article/CJFDTOTAL-GXKZ201901005.htmLI Chuangdi, ZHANG Yi, GE Xinguang. Equivalent damping of energy dissipating SDOF structure with Maxwell dampers based on spectral moment of frequency response function [J]. Journal of Guangxi University (Natural Science Edition), 2019, 44(1): 41-51. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GXKZ201901005.htm [22] COROTIS R B, VANMARCKE E H, CORNELL A C. First passage of nonstationary random processes[J]. Journal of Engineering Mechanics Division, 1972, 98(2): 401-414. [23] 方同. 工程随机振动[M]. 北京: 国防工业出版社, 1995.FANG Tong. Engineering Random Vibration[M]. Beijing: National Defense Industry Press, 1995. (in Chinese) [24] 林家浩. 随机振动的虚拟激励法[M]. 北京: 科学出版社, 2004.LIN Jiahao. Pseudo Excitation Method for Random Vibration[M]. Beijing: Science Press, 2004. (in Chinese) -





 下载:
下载:











 渝公网安备50010802005915号
渝公网安备50010802005915号